Template:Energy contour and preliminaries

Based on the method used in Evans and Davies 1968, a check can be made to ensure the solutions energy balance. The energy balance equation is derived by applying Green's theorem to [math]\displaystyle{ \phi }[/math] and its conjugate. The domain of integration is shown in the figure on the right.

A diagram depicting the area [math]\displaystyle{ \mathcal{U} }[/math] which is bounded by the rectangle [math]\displaystyle{ \mathcal{S} }[/math].] {A diagram depicting the area [math]\displaystyle{ \mathcal{U} }[/math] which is bounded by the rectangle [math]\displaystyle{ \mathcal{S} }[/math]. The rectangle [math]\displaystyle{ \mathcal{S} }[/math] is bounded by [math]\displaystyle{ -h\leq z \leq0 }[/math] and [math]\displaystyle{ -\infty\leq x \leq \infty }[/math]

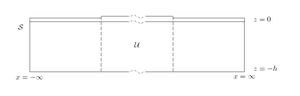
Applying Green's theorem to [math]\displaystyle{ \phi }[/math] and its conjugate [math]\displaystyle{ \phi^* }[/math] gives

[math]\displaystyle{ { \int\int_\mathcal{U}(\phi\nabla^2\phi^* - \phi^*\nabla^2\phi)dxdz = \int_\mathcal{S}(\phi\frac{\partial\phi^*}{\partial n} - \phi^*\frac{\partial\phi}{\partial n})dl }, }[/math]

where [math]\displaystyle{ n }[/math] denotes the outward plane normal to the boundary and [math]\displaystyle{ l }[/math] denotes the plane parallel to the boundary. As [math]\displaystyle{ \phi }[/math] and [math]\displaystyle{ \phi^* }[/math] satisfy the Laplace's equation, the left hand side of the Green theorem equation vanishes so that it reduces to

[math]\displaystyle{ \Im\int_\mathcal{S}\phi\frac{\partial\phi^*}{\partial n} dl = 0, }[/math]