Floating Elastic Plates of Identical Properties

Introduction

We begin by presenting the solution for the case of a single crack with waves incident from normal. The solution method is derived from Squire and Dixon 2001 and Evans and Porter 2005.

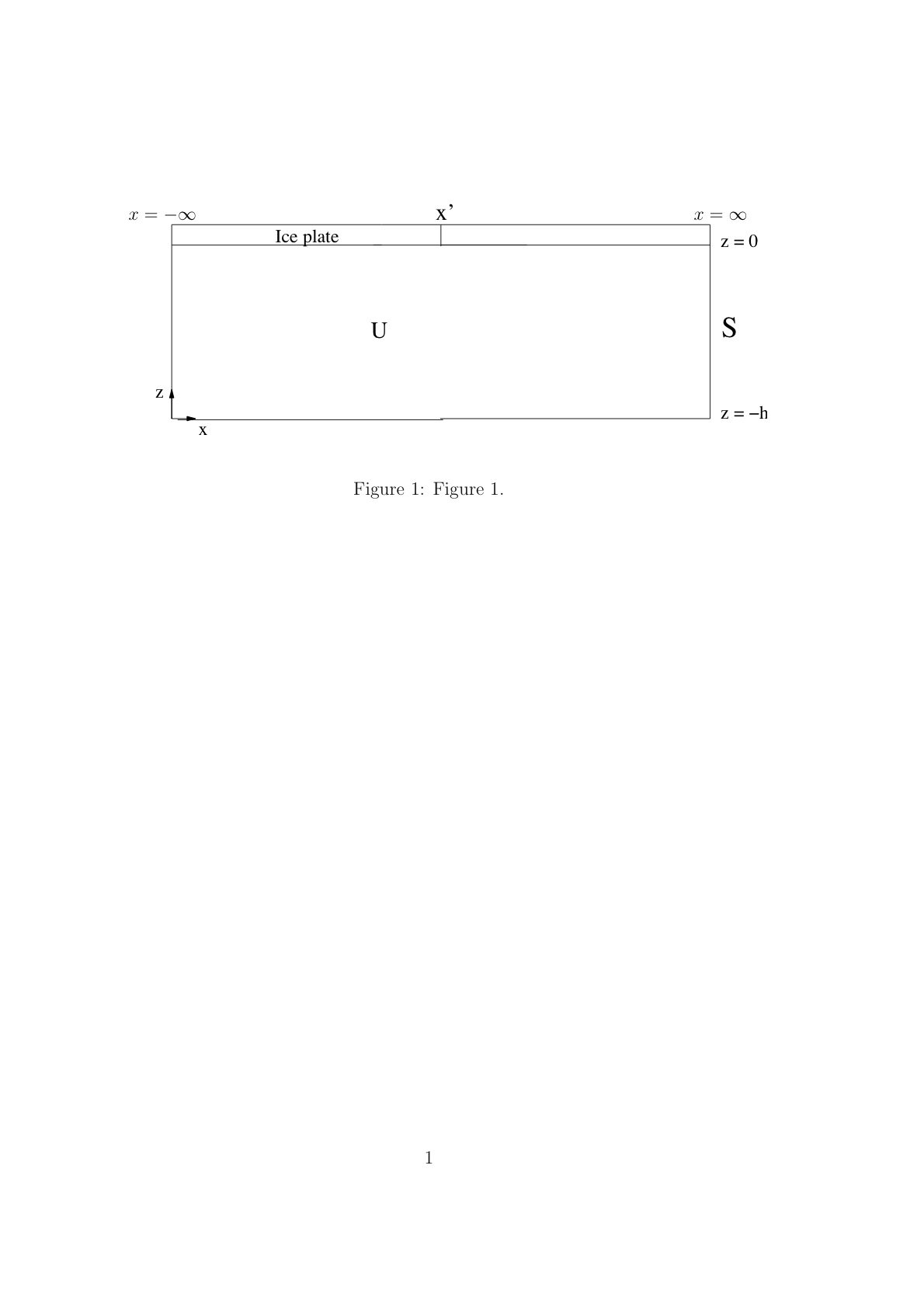
We consider the entire free surface to be occupied by a Floating Elastic Plate with a single discontinuity at [math]\displaystyle{ x=x^\prime }[/math] (Fig. 1).

The governing equations are

[math]\displaystyle{ \nabla^2 \phi(x,z) = 0, -H\lt z\lt 0, }[/math] [math]\displaystyle{ \frac{\partial \phi(x,z)}{\partial z} =0, z=-H, }[/math] [math]\displaystyle{ {\left( \beta \frac{\partial^4}{\partial x^4} - \gamma\alpha + 1\right)\frac{\partial \phi(x,z)}{\partial z} - \alpha \phi(x,z)} = 0, z=0, x\neq x^\prime. }[/math]

The Free-Surface Green Function for a Floating Elastic Plate satisfies the following equations (plus the Sommerfeld Radiation Condition far from the body)

[math]\displaystyle{ \nabla^2 G = 0, -H\lt z\lt 0, }[/math] [math]\displaystyle{ \frac{\partial G}{\partial z} =0, z=-H, }[/math] [math]\displaystyle{ {\left( \beta \frac{\partial^4}{\partial x^4} - \gamma\alpha + 1\right)\frac{\partial G}{\partial z} - \alpha G} = \delta(x-x^{\prime}), z=0, }[/math]

where

[math]\displaystyle{ G(x,x^{\prime},z) = -i\sum_{n=-2}^\infty\frac{\sin{(k_n H)}\cos{(k_n(z+H))}}{2\alpha C(k_n)}e^{-k_n|x-x^{\prime}|}, }[/math] [math]\displaystyle{ C(k_n)=\frac{1}{2}\left(h - \frac{(5\beta k_n^4 + 1 - \alpha\gamma)\sin^2{(k_n H)}}{\alpha}\right), }[/math]

and [math]\displaystyle{ k_n }[/math] are the solutions of the Dispersion Relation for a Floating Elastic Plate,

[math]\displaystyle{ \beta k^5 \sin(kH) - k \left(1 - \alpha \gamma \right) \sin(kH) = -\alpha \cos(kH) \, }[/math]

with [math]\displaystyle{ n=-1,-2 }[/math] corresponding to the complex solutions with positive real part, [math]\displaystyle{ n=0 }[/math] corresponding to the imaginary solution with negative imaginary part and [math]\displaystyle{ n\gt 0 }[/math] corresponding to the real solutions with positive real part.

Green's Second Identity

Since φ and G are both twice continuously differentiable on U, where U represents the area bounded by the contour, S (Fig 1), the Green's second identity can be applied and gives

[math]\displaystyle{ \int_U \left( G \nabla^2 \phi - \phi \nabla^2 G\right)\, dV = \oint_{\partial U} \left( G {\partial \phi \over \partial n} - \phi {\partial G \over \partial n}\right)\, dS }[/math]

where n repressents the plane normal to the boundary, S.

Our governing equations for G and [math]\displaystyle{ \phi }[/math] imply that the L.H.S of Green's second identity is zero so that

[math]\displaystyle{ 0 = \oint_{\partial U} \left( G {\partial \phi \over \partial n} - \phi {\partial G \over \partial n}\right)\, dS }[/math]

expanding gives

[math]\displaystyle{ 0 = -\int_{-\infty}^\infty \left( G {\partial \phi \over \partial z}|_{z=0} - \phi {\partial G \over \partial z}|_{z=0}\right)\, dx +\int_{-h}^0 \left( G {\partial \phi \over \partial x}|_{x=\infty} - \phi {\partial G \over \partial x}|_{x=\infty}\right)\, dz }[/math] [math]\displaystyle{ +\int_{-\infty}^\infty \left( G {\partial \phi \over \partial z}|_{z=-h} - \phi {\partial G \over \partial z}|_{z=-h}\right)\, dx -\int_{-h}^0 \left( G {\partial \phi \over \partial x}|_{x=-\infty} - \phi {\partial G \over \partial x}|_{x=-\infty}\right)\, dz }[/math]

Our governing equations imply [math]\displaystyle{ G {\partial \phi \over \partial z}|_{z=-h} = 0 }[/math] and [math]\displaystyle{ \phi {\partial G \over \partial z}|_{z=-h} = 0 }[/math] so that,

[math]\displaystyle{ 0 = -\int_{-\infty}^\infty \left( G {\partial \phi \over \partial z}|_{z=0} - \phi {\partial G \over \partial z}|_{z=0}\right)\, dx +\int_{-h}^0 \left( G {\partial \phi \over \partial x}|_{x=\infty} - \phi {\partial G \over \partial x}|_{x=\infty}\right)\, dz -\int_{-h}^0 \left( G {\partial \phi \over \partial x}|_{x=-\infty} - \phi {\partial G \over \partial x}|_{x=-\infty}\right)\, dz }[/math]

The vertical integrals at [math]\displaystyle{ x = \infty }[/math] and [math]\displaystyle{ x = -\infty }[/math] give the contribution [math]\displaystyle{ \phi_n^{In} }[/math] and we are left with

[math]\displaystyle{ 0 = -\int_{-\infty}^\infty \left( G(x,x^\prime,z) \phi_z(x,z)|_{z=0} - \phi(x,z) G_z(x,x^\prime,z) |_{z=0}\right)\, dx }[/math]

At z=0, the z variable disappears to give

[math]\displaystyle{ 0 = -\int_{-\infty}^\infty \left( G(x,x^\prime) \phi_z(x) - \phi(x) G_z(x,x^\prime)\right)\, dx }[/math]

We then substitute [math]\displaystyle{ G = \phi }[/math] to obtain

[math]\displaystyle{ \int_{-\infty}^{\infty}\left( G_{z}\left( x,x^{\prime }\right) \frac{1}{\alpha}\left( \beta \partial_{x^\prime}^4 -\gamma\alpha + 1\right)\phi_{z}( x) -G\left( x,x^{\prime }\right) \phi_{z}(x) \right) dx = 0 }[/math]

plus