Ship Kelvin Wake

Introduction

Wake created behind a ship

A ship moving over the surface of undisturbed water sets up waves emanating from the bow and stern of the ship. The waves created by the ship consist of divergent and transverse waves. The divergent wave are observed as the wake of a ship with a series of diagonal or oblique crests moving outwardly from the point of disturbance. These wave were first studies by Lord Kelvin

Kelvin wake

Diagram of the Kelvin Wake

Local view of Kelvin wake consists approximately of a plane progressive wave group propagating in direction [math]\displaystyle{ \theta\, }[/math]. As noted above surface wave systems of general form always consist of combinations of plane progressive waves of different frequencies and directions. The same model will apply to the ship kelvin wake. Relative to the earth frame, the local plane progressive wave takes the form:

[math]\displaystyle{ \Phi = \frac{igA}{\omega} e^{KZ-iK(X\cos\theta+Y\sin\theta)+i\omega t} }[/math]

Substitute

[math]\displaystyle{ X = x + Ut, Y=y, Z=z. \, }[/math]

and use the results from Translating Coordinate Systems to give us, relative to the ship frame

[math]\displaystyle{ \phi = \frac{igA}{\omega} e^{KZ-iK(x\cos\theta+y\sin\theta)-i(kU\cos\theta-\omega)t} }[/math]

But relative to the ship frame waves are stationary, so we must have:

[math]\displaystyle{ KU\cos\theta = \omega \, }[/math]

or

[math]\displaystyle{ \frac{\omega}{K} = V_P = U \cos \theta \, }[/math]

This implies the following

• The phase velocity of the waves in the kelvin wake propagating in direction [math]\displaystyle{ \theta\, }[/math] must be equal to [math]\displaystyle{ U\cos\theta\, }[/math], otherwise they cannot be stationary relative to the ship.
• Relative to the earth system the frequency of a local system propagating in direction [math]\displaystyle{ \theta \, }[/math] is given by the relation

[math]\displaystyle{ \omega = K U \cos \theta \, }[/math]

where [math]\displaystyle{ K = \frac{2\pi}{\lambda}\, }[/math].

Relative to the earth system the deep water dispersion relation states:

[math]\displaystyle{ \omega^2 = g K \, }[/math]

or

[math]\displaystyle{ K^2 U^2 \cos^2 \theta = g K \, }[/math] [math]\displaystyle{ \Longrightarrow K = \frac{g}{U^2 \cos^2 \theta} = \frac{2\pi}{\lambda(\theta)} }[/math] [math]\displaystyle{ \Longrightarrow \lambda(\theta) = \frac{2\pi U^2 \cos^2 \theta}{g} \, }[/math]

This is the wavelength of waves in a kelvin wake propagating in direction [math]\displaystyle{ \theta \, }[/math] and being stationary relative to the ship.

Application of the Group velocity

An observer sitting on an earth fixed frame observes a local wave system propagating in direction [math]\displaystyle{ \theta\, }[/math] travelling at its group velocity [math]\displaystyle{ \frac{d\omega}{dK}\, }[/math] by virtue of the Rayleigh device which states that we need to focus on the speed of the energy density ([math]\displaystyle{ \sim \, }[/math] wave amplitude) rather than the speed of wave crests. So, relative to the earth fixed inclined coordinate system [math]\displaystyle{ (x', y') \, }[/math]:

[math]\displaystyle{ \frac{x'}{t} = V_g = \frac{d\omega}{dK} \, }[/math]

Or

[math]\displaystyle{ X' = \frac{d\omega}{dK} t \ \Longrightarrow \ \frac{d}{dK} (K x' - \omega t) = 0 }[/math] [math]\displaystyle{ X' = X \cos \theta + Y \sin \theta = x \cos\theta + y\sin\theta + Ut \cos\theta \, }[/math]

So:

[math]\displaystyle{ Kx' - \omega t = K ( x\cos\theta + y\sin\theta ) + (KU\cos\theta - \omega) t \, }[/math]

However [math]\displaystyle{ (KU\cos\theta - \omega) t =0 }[/math] so that the Rayleigh condition for the velocity of the group takes the form:

[math]\displaystyle{ \frac{d}{dK} [ K(\theta) (x\cos\theta + y\sin\theta) ] = 0 \, }[/math]

By virtue of the dispersion relation derived above:

[math]\displaystyle{ K(\theta) = \frac{g}{U^2 \cos^\theta} \, }[/math]

It follows from the chain rule of differentiation that Rayleigh's condition is:

[math]\displaystyle{ \frac{d}{d\theta} \left[ \frac{g}{U^2\cos^2\theta} (x\cos\theta + y\sin\theta) ]\right] = 0 \, }[/math]

At the position of the Kelvin waves which are locally observed by an observer at the beach.

• So the "visible" waves in the wake of a ship are wave groups which must travel at the local group velocity. These conditions translate into the above equation which will be solved and discussed next. More discussion and a more mathematical derivation based on the principle of stationary phase can be found in Newman 1977.

Solution of the equation for angle

Graphical image of the equations

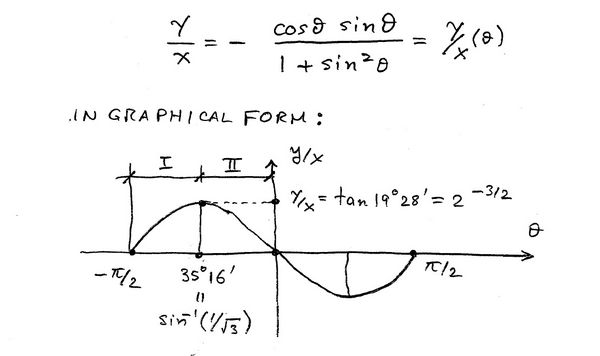
The solution of the above equation will produce a relation between [math]\displaystyle{ \frac{y}{x} \, }[/math] and [math]\displaystyle{ \theta\, }[/math]. So local waves in a Kelvin wake can only propagate in a certain direction [math]\displaystyle{ \theta\, }[/math], given [math]\displaystyle{ \frac{y}{x} \, }[/math].

Simple algebra leads to:

[math]\displaystyle{ \frac{y}{x} = - \frac{\cos\theta\sin\theta}{1+sin^2\theta} = \frac{y}{x}(\theta) }[/math]

which implies that

• [math]\displaystyle{ \frac{y}{x}(\theta) \, }[/math] is anti-symmetric about [math]\displaystyle{ \theta = 0 \, }[/math] eand ach part corresponds to the Kelvin wake in the port and starboard sides of the vessel. The physics on

either side is identical due to symmetry.

• [math]\displaystyle{ \theta = 0 \, }[/math]: waves propagating in the same direction as the ship. These waves can only exist at [math]\displaystyle{ Y = 0\, }[/math] as seen above.

• [math]\displaystyle{ \theta = \frac{\pi}{2} \, }[/math]: waves propagating at a [math]\displaystyle{ 90^\circ\, }[/math] angle relative to the ship direction of forward translation.

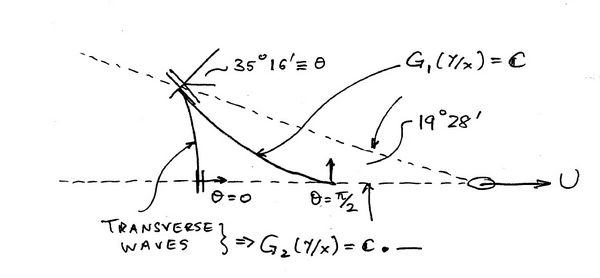
• [math]\displaystyle{ \theta = 35^\circ 16' \, }[/math]: waves propagating at an angle [math]\displaystyle{ \theta = 35^\circ 16' \, }[/math] relative to the ship axis. These are waves seen at the caustic of the Kelvin wake.

Let the solution of the [math]\displaystyle{ \frac{y}{x}\theta \, }[/math] be of the form, when inverted:

[math]\displaystyle{ \mbox{Region I}: \qquad \theta = f_1 (\frac{y}{x}) \, }[/math] [math]\displaystyle{ \mbox{Region II}: \qquad \theta = f_2 (\frac{y}{x}) \, }[/math]

Note that observable waves cannot exist for values of [math]\displaystyle{ \frac{y}{x}\, }[/math] that exceed the value shown in the figure or [math]\displaystyle{ \left. \frac{y}{x} \right|_{Max} = 2^{-3/2} \, }[/math]. This translates into a value for the corresponding angle equal to [math]\displaystyle{ 19^\circ 28' \, }[/math] which is the angle of the caustic for any speed [math]\displaystyle{ U\, }[/math].

"transverse" and "divergent" wave systems in the Kelvin wake

The crests of the wave system trailing a ship, the Kelvin wake, are curves of constant phase of:

[math]\displaystyle{ \frac{x\cos\theta+y\sin\theta}{\cos^2\theta} = \mathbf{C} \, }[/math]

In [math]\displaystyle{ \mbox{Region I} \, }[/math]:

[math]\displaystyle{ \mathbf{C} = \frac{x\cos f_1(\frac{y}{x} + y\sin f_1 (\frac{y}{x})}{\cos^2 f_1 (\frac{y}{x})} \equiv G_1 (\frac{y}{x}) }[/math]

In [math]\displaystyle{ \mbox{Region II} \, }[/math]:

[math]\displaystyle{ \mathbf{C} = \frac{x\cos f_2(\frac{y}{x} + y\sin f_2 (\frac{y}{x})}{\cos^2 f_2 (\frac{y}{x})} \equiv G_2 (\frac{y}{x}) }[/math]

Plotting these curves we obtain a visual graph of the "transverse" and "divergent" wave systems in the Kelvin wake.

---

This article is based on the MIT open course notes and the original article can be found here

Ocean Wave Interaction with Ships and Offshore Energy Systems