Wavemaker Theory

Introduction

Wavemaker

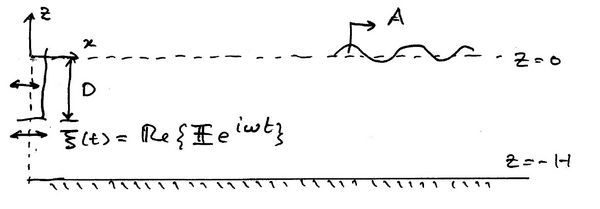
We will derive the potential in a two-dimensional wavetank due the motion of the wavemaker. The method is based on the Eigenfunction Matching Method. A paddle with draft [math]\displaystyle{ D\, }[/math] is undergoing small amplitude horizontal oscillations with displacement

[math]\displaystyle{ \xi (t) = \mathfrak{Re} \left \{ f(z) e^{i\omega t} \right \} }[/math]

where [math]\displaystyle{ f(z) }[/math] is assumed known. Since the time [math]\displaystyle{ t=0 }[/math] is arbitrary we can assume that [math]\displaystyle{ f(z) }[/math] is real but this is not necessary. Because the oscillations are small the linear equations apply (which will be given formally below). This excitation creates plane progressive waves with amplitude [math]\displaystyle{ A \, }[/math] down the tank. The principal objective of wavemaker theory is to determine [math]\displaystyle{ A \, }[/math] as a function of [math]\displaystyle{ \omega, f(z) \, }[/math] and [math]\displaystyle{ H \, }[/math]. Time-dependent wavemaker theories can also be developed.

Expansion of the solution

In general, the wavemaker displacement at [math]\displaystyle{ X=0\, }[/math] may be written in the form

[math]\displaystyle{ \xi(t) = \mathfrak{Re} \left \{ f (z) e^{i\omega t} \right \} }[/math]

where [math]\displaystyle{ f(z) \, }[/math] is a known function of [math]\displaystyle{ z \, }[/math]. The standard linear equations apply. Let the total velocity potential be

[math]\displaystyle{ \Phi(x,z,t) = \mathfrak{Re} \left \{ \phi(x,z) e^{i\omega t} \right \} }[/math]

.

This gives us a Frequency Domain Problem. The water is assumed to have constant finite depth [math]\displaystyle{ H }[/math] and the [math]\displaystyle{ z }[/math]-direction points vertically upward with the water surface at [math]\displaystyle{ z=0 }[/math] and the sea floor at [math]\displaystyle{ z=-H }[/math]. The boundary value problem can therefore be expressed as

[math]\displaystyle{ \Delta\phi=0, \,\, -H\lt z\lt 0, }[/math]

[math]\displaystyle{ \phi_{z}=0, \,\, z=-H, }[/math]

[math]\displaystyle{ \partial_z\phi=\alpha\phi, \,\, z=0,\,x\lt 0, }[/math]

[math]\displaystyle{ \partial_x\phi= i\omega f(z), \,\,x=0. }[/math]

We must also apply the Sommerfeld Radiation Condition as [math]\displaystyle{ x\rightarrow\infty }[/math]. This essentially implies that the only wave at infinity is propagating away.

Separation of variables

We now separate variables and write the potential as

[math]\displaystyle{ \phi(x,z)=\zeta(z)\rho(x) }[/math]

Applying Laplace's equation we obtain

[math]\displaystyle{ \zeta_{zz}+k^{2}\zeta=0. }[/math]

We then use the boundary condition at [math]\displaystyle{ z=-H }[/math] to write

[math]\displaystyle{ \zeta=\cos k(z+H) }[/math]

The boundary condition at the free surface ([math]\displaystyle{ z=0 }[/math]) is

[math]\displaystyle{ k\tan\left( kH\right) =-\alpha,\quad x\lt 0 }[/math]

which is the Dispersion Relation for a Free Surface We denote the positive imaginary solution of this equation by [math]\displaystyle{ k_{0} }[/math] and the positive real solutions by [math]\displaystyle{ k_{m} }[/math], [math]\displaystyle{ m\geq1 }[/math]. We define

[math]\displaystyle{ \phi_{m}\left( z\right) =\frac{\cos k_{m}(z+H)}{\cos k_{m}H},\quad m\geq0 }[/math]

as the vertical eigenfunction of the potential in the open water region. From Sturm-Liouville theory the vertical eigenfunctions are orthogonal. They can be normalised to be orthonormal, but this has no advantages for a numerical implementation. It can be shown that

[math]\displaystyle{ \int\nolimits_{-H}^{0}\phi_{m}(z)\phi_{n}(z) d z=A_{n}\delta_{mn} }[/math]

where

[math]\displaystyle{ A_{n}=\frac{1}{2}\left( \frac{\cos k_{n}H\sin k_{m}H+k_{n}H}{k_{n}\cos ^{2}k_{n}H}\right) }[/math]

and

Expansion in Eigenfunctions

The wavemaker velocity potential [math]\displaystyle{ \phi }[/math] can be expressed simply in terms of eigenfunctions

[math]\displaystyle{ \phi = \sum_{n=0}^{\infty} a_n \phi_n (x) e^{-k_n x} }[/math]

and we can solve for the coefficients by matching at [math]\displaystyle{ x=0 }[/math]

[math]\displaystyle{ \left. \phi_x(z) \right|_{x=0} = \sum_{n=0}^{\infty} -k_n a_n \phi_n (x) e^{-k_n x} = i\omega f(z) }[/math]

It follows that

[math]\displaystyle{ a_n = -\frac{ i \omega}{k_n} A_n }[/math]

Far Field Wave

One of the primary objecives of wavemaker theory is to determine the far-field wave amplitude [math]\displaystyle{ A \, }[/math] ) in terms of [math]\displaystyle{ f(z) }[/math]. The far-field wave component representing progagating waves is given by:

[math]\displaystyle{ \phi_w = a_0 \frac{\cos k_0(z-h)}{\cos k_0 H } e^{-ik_0 x} }[/math]

We therefore obtain the complex amplitude of the propagating wave at infinity, namely modulus and phase, in terms of the wave maker displacement [math]\displaystyle{ f(z) }[/math].

For what type of [math]\displaystyle{ f(z) }[/math] are the non-wavelike modes zero? It is easy to verify by virtue of orthogonality that

[math]\displaystyle{ f(z) \ \sim \ \phi_0 (z) }[/math]

Unfortunately this is not a "practical" displacement since [math]\displaystyle{ \phi_0 (z) \, }[/math] depends on [math]\displaystyle{ \omega\, }[/math]. So one would need to build a flexible paddle.

---

This article is based on the MIT open course notes and the original article can be found here

Ocean Wave Interaction with Ships and Offshore Energy Systems