Floating Elastic Plates of Identical Properties

Introduction

We begin by presenting the solution for the case of a single crack with waves incident from normal. The solution method is derived from Squire and Dixon 2001 and Evans and Porter 2005.

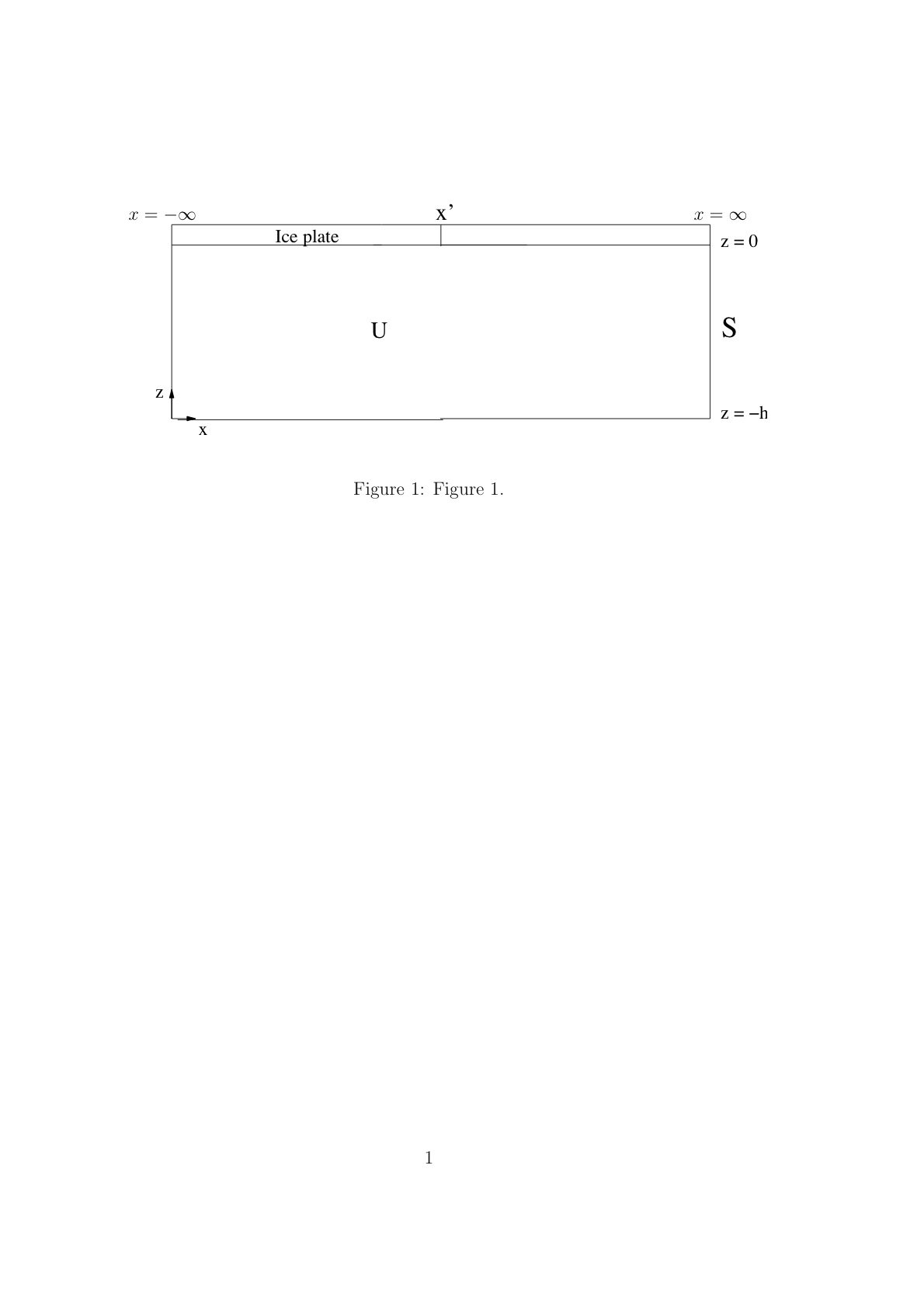
We consider the entire free surface to be occupied by a Floating Elastic Plate with a single discontinuity at [math]\displaystyle{ x=x^\prime }[/math] (Fig. 1).

The governing equations are

[math]\displaystyle{ \nabla^2 \phi(x,z) = 0, -H\lt z\lt 0, }[/math] [math]\displaystyle{ \frac{\partial \phi(x,z)}{\partial z} =0, z=-H, }[/math] [math]\displaystyle{ {\left( \beta \frac{\partial^4}{\partial x^4} - \gamma\alpha + 1\right)\frac{\partial \phi(x,z)}{\partial z} - \alpha \phi(x,z)} = 0, z=0, x\neq x^\prime. }[/math]

The Free-Surface Green Function for a Floating Elastic Plate satisfies the following equations (plus the Sommerfeld Radiation Condition far from the body)

[math]\displaystyle{ \nabla^2 G = 0, -H\lt z\lt 0, }[/math] [math]\displaystyle{ \frac{\partial G}{\partial z} =0, z=-H, }[/math] [math]\displaystyle{ {\left( \beta \frac{\partial^4}{\partial x^4} - \gamma\alpha + 1\right)\frac{\partial G}{\partial z} - \alpha G} = \delta(x-x^{\prime}), z=0, }[/math]

where

[math]\displaystyle{ G(x,x^{\prime},z) = -i\sum_{n=-2}^\infty\frac{\sin{(k_n H)}\cos{(k_n(z+H))}}{2\alpha C(k_n)}e^{-k_n|x-x^{\prime}|}, }[/math] [math]\displaystyle{ C(k_n)=\frac{1}{2}\left(h - \frac{(5\beta k_n^4 + 1 - \alpha\gamma)\sin^2{(k_n H)}}{\alpha}\right), }[/math]

and [math]\displaystyle{ k_n }[/math] are the solutions of the Dispersion Relation for a Floating Elastic Plate,

[math]\displaystyle{ \beta k^5 \sin(kH) - k \left(1 - \alpha \gamma \right) \sin(kH) = -\alpha \cos(kH) \, }[/math]

with [math]\displaystyle{ n=-1,-2 }[/math] corresponding to the complex solutions with positive real part, [math]\displaystyle{ n=0 }[/math] corresponding to the imaginary solution with negative imaginary part and [math]\displaystyle{ n\gt 0 }[/math] corresponding to the real solutions with positive real part.

---

????????????????? My imaginary solution has a negative imaginary real part rather than a +ve imaginary real part ?????????????

---

Green's Second Identity

Since φ and G are both twice continuously differentiable on U, where U represents the area bounded by the contour, S (Fig 1), the Green's second identity can be applied and gives

[math]\displaystyle{ \int_U \left( G \nabla^2 \phi - \phi \nabla^2 G\right)\, dV = \oint_{\partial U} \left( G {\partial \phi \over \partial n} - \phi {\partial G \over \partial n}\right)\, dS }[/math]

where n repressents the plane normal to the boundary, S.

Our governing equations for G and [math]\displaystyle{ \phi }[/math] imply that the L.H.S of Green's second identity is zero so that

[math]\displaystyle{ 0 = \oint_{\partial U} \left( G {\partial \phi \over \partial n} - \phi {\partial G \over \partial n}\right)\, dS }[/math]

expanding gives [math]\displaystyle{ 0 = -\int_{-\infty}^\infty \left( G {\partial \phi \over \partial z}|_{z=0} - \phi {\partial G \over \partial z}|_{z=0}\right)\, dx +\int_{-h}^0 \left( G {\partial \phi \over \partial x}|_{x=\infty} - \phi {\partial G \over \partial x}|_{x=\infty}\right)\, dz }[/math]

[math]\displaystyle{ +\int_{-\infty}^\infty \left( G {\partial \phi \over \partial z}|_{z=-h} - \phi {\partial G \over \partial z}|_{z=-h}\right)\, dx -\int_{-h}^0 \left( G {\partial \phi \over \partial x}|_{x=-\infty} - \phi {\partial G \over \partial x}|_{x=-\infty}\right)\, dz }[/math]

---

???????????? Mike, can you please check my +ve's and -ve's above. I assumed we are integrating anti-clockwise. ?????????????

---

Our governing equations imply [math]\displaystyle{ G {\partial \phi \over \partial z}|_{z=-h} = 0 }[/math] and [math]\displaystyle{ \phi {\partial G \over \partial z}|_{z=-h} = 0 }[/math] so that,

[math]\displaystyle{ 0 = -\int_{-\infty}^\infty \left( G {\partial \phi \over \partial z}|_{z=0} - \phi {\partial G \over \partial z}|_{z=0}\right)\, dx +\int_{-h}^0 \left( G {\partial \phi \over \partial x}|_{x=\infty} - \phi {\partial G \over \partial x}|_{x=\infty}\right)\, dz -\int_{-h}^0 \left( G {\partial \phi \over \partial x}|_{x=-\infty} - \phi {\partial G \over \partial x}|_{x=-\infty}\right)\, dz }[/math]

The vertical integrals at [math]\displaystyle{ x = \infty }[/math] and [math]\displaystyle{ x = -\infty }[/math] give the contribution [math]\displaystyle{ \phi_n^{In} }[/math] and we are left with

[math]\displaystyle{ 0 = -\int_{-\infty}^\infty \left( G(x,x^\prime,z) \phi_z(x,z)|_{z=0} - \phi(x,z) G_z(x,x^\prime,z) |_{z=0}\right)\, dx }[/math]

---

???????????? Don't fully understand the above. ?????????????

---

At z=0, the z variable disappears to give

[math]\displaystyle{ 0 = -\int_{-\infty}^\infty \left( G(x,x^\prime) \phi_z(x) - \phi(x) G_z(x,x^\prime)\right)\, dx }[/math]

We then substitute [math]\displaystyle{ G = \phi }[/math] to remove [math]\displaystyle{ \phi }[/math] and obtain

[math]\displaystyle{ \int_{-\infty}^{\infty}\left( G_{z}\left( x,x^{\prime }\right) \frac{1}{\alpha}\left( \beta \partial_{x}^4 -\gamma\alpha + 1\right)\phi_{z}( x) -G\left( x,x^{\prime }\right) \phi_{z}(x) \right) dx = 0 }[/math]

We now integrate by parts remembering that [math]\displaystyle{ \phi_z }[/math] is continuous everywhere except at [math]\displaystyle{ x = x^\prime }[/math] so that

[math]\displaystyle{ \int_{-\infty}^\infty(\partial_x^4\phi_z)G_z dx = \int_{-\infty}^{x^\prime}(\partial_x^4\phi_z)G_z dx + \int_{x^\prime}^\infty(\partial_x^4\phi_z)G_z dx }[/math]

and obtain

[math]\displaystyle{ \int_{-\infty}^{\infty}\left\{ \frac{1}{\alpha}\left( \beta \partial_{x}^4 - \gamma\alpha + 1\right)G_{z}\left( x,x^{\prime }\right) - G( x,x^\prime)\right\} \phi_z(x)dx }[/math]

[math]\displaystyle{ - \frac{\beta}{\alpha}\left(-\partial_{x}^3G_z(x,x^\prime)[\phi_z] + \partial_{x}^2 G_z(x,x^\prime)\partial_x[\phi_z] - \partial_{x} G_z(x,x^\prime)\partial_{x}^2[\phi_z] + G_z(x,x^\prime)\partial_{x}^3[\phi_z] \right) =0 }[/math]

where [] denotes the jump in the function at [math]\displaystyle{ x = x^{\prime} }[/math].

---

??????????? Note that above I have taken the opposite sign to what was used previously. It is not obvious to me what the sign should be ??????????

---

The integral can be simplified using the delta function property of the Green function to give us

[math]\displaystyle{ \phi_{z}\left( x\right) = -\beta\left(\partial_{x}^3 G_z [\phi_z] - \partial_{x}^2 G_z [\partial_{x}\phi_z] + \partial_{x} G_z [\partial_{x}^2\phi_z] - G_z [\partial_{x}^3\phi_z]\right) }[/math]

We can write the equation in terms of [math]\displaystyle{ \phi }[/math] as was done by Porter and Evans 2005 but there is no real point because the boundary conditions are given in terms of [math]\displaystyle{ \phi_z }[/math] since this represents the displacement.

We include the boundary conditions at infinity, which we omitted earlier, to give the full equation

[math]\displaystyle{ \phi_{z}(x,z) = \phi_z^\mathrm{In} - \beta(\partial_x^3 G_z[\phi_z] - \partial_{x}^2 G_z[\partial_{x}\phi_z] + \partial_{x} G_z [\partial_{x}^2\phi_z] - G_z [\partial_{x}^3\phi_z]) }[/math]

which can be solved by applying the edge conditions at [math]\displaystyle{ x=x^\prime }[/math] and z = 0

[math]\displaystyle{ \partial_x^2\phi_z=0,\,\,\, {\rm and}\,\,\,\, \partial_x^3\phi_z=0. }[/math]

Solution

We re-express [math]\displaystyle{ \phi_z }[/math] as

[math]\displaystyle{ \phi_{z} = \phi_{z}^{\mathrm{In}} + \psi_a [\phi_z] - \psi_s [\partial_{x}\phi_z] + \chi_a[\partial_{x}^2\phi_z] - \chi_s [\partial_{x}^3\phi_z] }[/math]

where

[math]\displaystyle{ \chi_s = \beta G_z = \frac{i\beta}{2\alpha} \sum_{n=-2}^\infty\frac{k_n\sin^2{(k_n H)}}{C(k_n)}e^{-k_n|x-x^\prime|}, }[/math] [math]\displaystyle{ \chi_a = \beta\partial_x G_z = -sgn(x-x^\prime)\frac{i\beta}{2\alpha} \sum_{n=-2}^\infty\frac{k_n^2\sin^2{(k_n H)}}{C(k_n)}e^{-k_n|x-x^\prime|}, }[/math] [math]\displaystyle{ \psi_s = \beta\partial_x^2 G_z = \frac{i\beta}{2\alpha} \sum_{n=-2}^\infty\frac{k_n^3\sin^2{(k_n H)}}{C(k_n)}e^{-k_n|x-x^\prime|}, }[/math] [math]\displaystyle{ \psi_a =\beta\partial_x^3 G_z = sgn(x-x^\prime)\frac{i\beta}{2\alpha} \sum_{n=-2}^\infty\frac{k_n^4\sin^2{(k_n H)}}{C(k_n)}e^{-k_n|x-x^\prime|} }[/math]

and

[math]\displaystyle{ \phi_z^{In} = e^{k_0x}\frac{\sin(k_0(z+H))}{\sin(k_0H)} }[/math]

The jump coefficients [math]\displaystyle{ [\phi_z], [\partial_{x}\phi_z], [\partial_{x}^2\phi_z] }[/math] and [math]\displaystyle{ [\partial_{x}^3\phi_z] }[/math] can be found by applying the edge conditions given above. The edge conditions imply that [math]\displaystyle{ [\partial_{x}^2\phi_z] }[/math] and [math]\displaystyle{ [\partial_{x}^3\phi_z] }[/math] are zero so that [math]\displaystyle{ \phi_z }[/math] becomes

[math]\displaystyle{ \phi_{z}( x) = \phi_{z}^{\mathrm{In}}(x) +\psi_a [\phi_z] - \psi_s [\partial_{x}\phi_z] }[/math]

We are now left with two unknowns which can be solved using the two edge conditions. To solve, we use

[math]\displaystyle{ \partial_x^2\phi_z = \partial_x^2\phi_z^{In} + \partial_x^2\psi_a [\phi_z] - \partial_x^2\psi_s [\partial_{x}\phi_z] }[/math]

[math]\displaystyle{ = k_0^2e^{k_0x} \frac{\sin(k_0z+H)}{\sin(k_0H)} + \frac{i\beta}{2\alpha}\sum_{n=-2}^\infty \frac{\sin^2{(k_n H)}}{ C(k_n)}e^{-k_n|x-x^\prime|} \left[sgn(x-x^\prime)k_n^6[\phi_z] - k_n^5[\partial_{x}\phi_z] \right] }[/math]

and

[math]\displaystyle{ \partial_x^3\phi_z = \partial_x^3\phi_z^{In} + \partial_x^3\psi_a [\phi_z] - \partial_x^3\psi_s [\partial_{x}\phi_z] }[/math]

[math]\displaystyle{ = k_0^3e^{k_0x} \frac{\sin(k_0z+H)}{\sin(k_0H)} + \frac{i\beta}{2\alpha}\sum_{n=-2}^\infty \frac{\sin^2{(k_n H)}}{ C(k_n)}e^{-k_n|x-x^\prime|} \left[-k_n^7[\phi_z] + sgn(x-x^\prime)k_n^6[\partial_{x}\phi_z] \right] }[/math]

At [math]\displaystyle{ x=x^\prime }[/math] and z=0, the first edge conditions gives

[math]\displaystyle{ k_0^2e^{k_0x^\prime} + \frac{i\beta}{2\alpha}\sum_{n=-2}^\infty \frac{\sin^2{(k_n H)}}{ C(k_n)} \left[sgn(x-x^\prime)k_n^6[\phi_z] - k_n^5[\partial_{x}\phi_z] \right] = 0 }[/math]

and the second edge condition gives

[math]\displaystyle{ k_0^3e^{k_0x^\prime} + \frac{i\beta}{2\alpha}\sum_{n=-2}^\infty \frac{\sin^2{(k_n H)}}{ C(k_n)} \left[-k_n^7[\phi_z] + sgn(x-x^\prime)k_n^6[\partial_{x}\phi_z] \right] = 0 }[/math]

The jump conditions [math]\displaystyle{ [\phi_z] }[/math] and [math]\displaystyle{ [\partial_{x}\phi_z] }[/math] can be solved by solving the edge conditions simultaneously.

The reflection and transmission coefficients, [math]\displaystyle{ R }[/math] and [math]\displaystyle{ T }[/math] can be found by taking the limit of [math]\displaystyle{ \phi_z }[/math] as [math]\displaystyle{ x\rightarrow\pm\infty }[/math] to obtain

[math]\displaystyle{ R = 1 - \frac{i\beta\sin^2(k_0h)}{2\alpha C(k_0)}\left[k_0^4[\phi_z] + k_0^3[\partial_{x}\phi_z]\right] }[/math]

and

[math]\displaystyle{ T= 1 + \frac{i\beta\sin^2(k_0h)}{2\alpha C(k_0)}\left[k_0^4[\phi_z] + k_0^3[\partial_{x}\phi_z]\right] }[/math]

---

??????????????

My code gets the correct solution for R if

[math]\displaystyle{ R = \frac{i\beta\sin^2(k_0h)}{2\alpha C(k_0)}\left[k_0^4[\phi_z] + k_0^3[\partial_{x}\phi_z]\right] }[/math]

Basically the negative of what I thought it should be. I know there shouldn't be a 1 for the incident wave, but I can't explain what happens to it

????????????????

---